Standards Adopted for the New World
In order to introduce the changes made with spelling, numeration, weights, measures, directions, the calendar, and timekeeping, the initial presentations, before any of these standards were adopted, have been reprinted here. For brevity and clarity, only the most important adopted standards have been included, and lesser but also important standards such as voltage, parts, bolts and nuts, and jar lid sizes have been excluded. Also excluded were standards which weren't accepted.
Proposed Standards for the New World
by Alice Hepfield
In September 1966 [year two, Aquarius], several meetings were held to discuss whether we should emigrate to the New World or not and also under what conditions. During one of those meetings, since I had first suggested the idea of establishing standards, the chairmanship of the standards committee was given to me. I immediately passed all the work on to my colleagues, choosing people who were knowledgeable in each area. I will now ask each of them to give a report of their opinions in order for you to discuss whether you wish to make such sweeping changes or not.
I have examined their findings, and I feel it is important, perhaps necessary, that I make some preliminary statements. I did not ask these people to come up with standards that would be the easiest and quickest to adopt. If we were going to do that, we might as well use the standard American systems that we already have or adopt the metric system. Such adaptations would call for little effort on our parts. However, in our community we have never chosen the broad easy way; instead we have looked for a better way of doing things than has been done before. Outsiders look at our choices, such as the spinning wheel, the hand loom, and hand-made clothing, and consider them foolish, but we would rather have warm, properly fitting, and well-designed clothing, even if that means that we have less. We don't mind being different from all the rest of the world if what we do makes sense.
Now we have a unique opportunity to start civilization all over again. Do we wish to begin by following the misguided footprints of our ancestors in every matter, or do we wish to create a better world, even if it means more difficulty initially? And in truth, creating many of these standards will save us work right from the beginning. For instance, currently bicycle wheels are produced in the following sizes: 20", 22", 24", 650mm, 26", 700mm, 27", and 28". In addition, not all tires of a given size are usable with all wheels of that size. Allowing only two or three wheel sizes would satisfy all of our needs and make producing wheels and tires much easier. As another instance, with bolt head sizes, there are twenty different sizes, English and metric, larger than half an inch and smaller than one inch. Is there any possible advantage for having 20 different sizes of bolt heads and wrenches where one would suffice?
In making the following recommendations, my colleagues' objectives were to make real improvements that would over the years result in a real advantage, even though immediately the advantage might be small, none, or even a disadvantage. However, I feel that changes that make us distinctive and help us identify with each other can be good, even if their other advantages do not show up immediately.
The math and spelling changes have been advanced at one time or another on a theoretical basis in our community, usually in our school, but we will explain each of them as if you are hearing it for the first time, as some of you undoubtably are.
Two of the changes, spelling reform and number base change, will be discussed first, as they will have the greatest impact on everything else, and many of the other decisions will depend on these.
I have asked Dorothy Johnson to begin by explaining spelling reform to us.
SPELLING REFORM
by Dorothy Johnson
Most of you were educated in our own school, so this will be basically a review of what you already know.
Of all the languages which use an alphabet, English is the least phonetic. A phonetic alphabet can be defined as one in which each letter has only one specific sound and every sound has one specific letter, so any unfamiliar word can be pronounced or spelled correctly. It has been estimated that only 50% of the words in English can be spelled following any set of rules; the rest must be memorized. However, how does one figure out which of the words follow the rules and which do not? And, who knows all of the rules? And if we know a rule, do we remember the entire rule and all the exceptions? Most people remember "i before e, except after c," but they are not likely to know that the rule also states that the "e" goes before "i" in words with a long "a" such as "neighbor" and "weight," and "i" goes before "e" after a "c" if the "c" is pronounced "sh" as in "ancient" and "efficient," and -- even if they do -- they still need to memorize a long list of exceptions, including "science," "foreign," "society," and "weird." Is there any advantage to having such a rule?
To give an example of how bad English can be, consider the following words: cough, tough, bough, through, though, and thorough. Although they all contain the same "ough," they are pronounced six different ways. The vowel sound in "ate" is found spelled 25 different ways, and the consonant sound found in "shoe" is spelled 14 different ways; in fact, most vowels and consonants can be spelled a variety of ways. As a result, the only way to spell words correctly in English is by memorizing them. We have several sounds in English, including the most common sound, that are not represented by any letter, and we have several letters in English that have no corresponding sound.
Here's a quote from Stuart Robinson, a language scholar and author of The Development of Modern English: "The fact is that English spelling has, in the course of time, become so unphonetic that, in order to learn to spell well, one has to make a positive effort not to try to connect the letters to single sounds. Since spelling gets a good deal of attention in the early school of most people, we are, in effect, trained to ignore or misapprehend the actual sounds of speech."
How did we get in such a mess? In the beginning, Anglo-Saxon scribes had to figure ways to use a foreign alphabet (the Roman), which did not have all of the sounds of their language. They added a few letters and did fairly well. After the Norman invasion, it was thought that English as a language would die out, since the Normans all spoke French. Books written in English were destroyed, and the teaching of English forbidden. When it finally was recognized that English was not going to disappear, and as educated people began to use it, it became necessary to be able to write the language again, but there was a problem of how to indicate sounds which did not exist in French. This was accomplished by using pairs of letters, usually involving an "h," as in "church," "enough," "shout," "thing" and "that," and "who," but sometimes using "n," such as "knight," "gnat," and "ring." Since then, we have lost some of these sounds, the "gh," "wh" (for most people), "kn," and "gn," but we still have the spelling. In addition, many words of Anglo-Saxon origin not using these sounds have been given other French or Latin spellings. Then Caxton, the first printer in England, introduced Dutch spellings for some words, giving us "ghost" among many others (the "h" was never pronounced). After that, there was the Great Vowel Shift in which most vowel sounds moved upward in the mouth (downward in the alphabet), but not all the vowels changed, which thoroughly confused the correspondence of vowels to letters. When educated people began to work on this muddle in the 1800's, they did more to make matters worse through their desire to use historical spellings, although often the words were unrelated to the words they were made to imitate, thus we get the "b" in "debt," the "s" in "island," the "t" in "often" and "mortgage," the "l' in "salmon," the "g" in "foreign," the "p" in "receipt," the "c" in "perfect," and many others, most of which are not pronounced by anyone. In the years that followed, we have imported hundreds of thousands of new words from every language, and we usually follow that language's spelling and pronunciation.
Many people support this babble. They give several reasons: 1. The history of the word is often contained within a spelling, even if the sound is inaccurate. Related words are spelled alike even if they now sound different. For instance, "telegraph" and "telegraphy" would no longer be next to one another in a phonetic dictionary. But, if this really is the reason for preserving these spellings, why do we spell "procedure" and "proceeding" differently? And "impertinence" and "maintenance" differently from each other and "maintenance" differently from "maintain"? English is full of closely-related words which are not spelled alike. 2. The eye can catch an unusual spelling much more quickly. "Knight" is a good example. While it lost its distinctive pronunciation over five hundred years ago, its distinctive spelling makes it easy to spot. But if this is the rule, why do we spell "bow" meaning part of a ship and "bow" meaning a weapon used with arrows the same, even though they are pronounced differently? They do share a common historical past, but is that helpful? 3. Readers have already had to invest an enormous amount of time memorizing the spelling of thousands of words; if we change the system, they'll have to start over again. That's very true, but should we force future generations to go through the same struggle forever just so one generation doesn't have to learn twice? One can learn a phonetic alphabet within a few months, but a lifetime is not long enough to memorize all the spellings of a non-phonetic one.
The problem of learning to spell in English is not trivial. 1. It makes it very hard for children to learn to read. Although English-speaking children spend more time on spelling than do children using any other language, the illiteracy rate is much higher than in equivalent countries using more phonetic alphabets. Even the Japanese have a higher literacy rate than we do, although their people must learn three systems, one of which consists of ideograms. 2. It makes it impossible to spell words you don't know, leaving their whereabouts in the dictionary unknown. 3. It makes it impossible to pronounce words you don't know unless you have a dictionary.
With a true phonetic alphabet, each sound has one letter and each letter has one sound. True phonetic alphabets are very accurate and are used by scholars to record variations in speech from one English speaking area to another. Unfortunately, a true phonetic alphabet also makes reading and writing slow, as the writer must carefully sound out each word. There's no practical reason for being this careful to record sounds in everyday writing and reading.
However, one can also create a phonemic alphabet. While the letters are much the same, a phonemic alphabet is less exact. It doesn't record exactly what the speaker is saying right now; it records instead the approximate sound of the word when it is spoken normally by the majority of people. Phonemic alphabets ignore regional variations that the speakers don't usually pay any attention to; thus practically all words can be spelled the same by all speakers in all contexts.
One desirable feature for a phonemic alphabet for English is that it should be easy to read by someone trained to read our current spelling. This is not an easy task, as the original spelling is so poor. Most people, when they find they can't read a different spelling, assume that the new spelling and not the old has a problem. A second desirable feature is for it to use the same letters as the old, since there are no additional letters on a typewriter. Two problems exist: 1. the old letters don't represent the language accurately and 2. the English language has more than twice as many vowel sounds as we have letters.
Assuming that we intend to make a phonemic alphabet for English, what letters would we have to change? Let's look first at the consonants.
In English we have four letters for consonants which don't make sense. "C" is pronounced /s/ or /k/, but we already have both of those letters, so we don't need it. "X" is pronounced /k/ or /z/, but we already have those letters, so we don't need it either. "Q" has the same sound as /k/ and therefore also is unnecessary. "J" is a letter which has been assigned a sound which is really a combination of two sounds, one of which we don't have a letter for. Also, "g" is sometimes used instead of "j" for the same sound.
On the other hand, English has a number of sounds which no single letter represents. This includes the first sounds of "that," "this," and "shoot," the sound common to "pleasure" and "garage," and the final sound in "ring." English also has two double consonants which are not correctly recognized, the first and last sounds in "church" and "judge."
There are letters used for these sounds by language scholars, but they are not found on the typewriter. As a solution for typewriter use, I suggest using the letter "q" for the first sounds of "this" and "that," the "c" for the first sound in "shoot," "x" for the last sound in "ring," "tc" for the first and last sound in "church," "j" for the last sound in "garage," and "dj" for the first and last sound in "judge."
The problem with vowels is that we have five letters but over twice that number of sounds, and the most important vowel sound is not represented by any letter.
The most important vowel sound is sometimes written as "uh." It occurs more often than any other sound in the English language, even though we have no letter to represent it. It is spelled fourteen different ways. Some words with this sound are "about," "ernest," "easily," "gallop," and "circus." I suggest, as a temporary measure, using the carat ("^") for this sound when using a typewriter, and using the phonetic letter, which is an upside down "e" otherwise.
Looking at the other vowel sounds in English, we find that we can approximate the sounds of the language with the five vowel sounds if we separate them according to the position that they are made within the mouth and then by their tenseness, with the less tense sound considered longer. This method of distinguishing the sounds should not be confused with the long and short sounds in Latin nor with the long and short vowel markings used by the dictionary. High and front in the mouth are vowel sounds in "bit" and "beet." I suggest that we use "i" for the first and "ii" or long "i" for the second. In the middle front of the mouth, we form the vowel sounds found in "bet" and "bait." I suggest "e" for the first, and "ei" for the second (the long "e" is always diphthongized in English; see below). Low and front in the mouth is the vowel sound found in "bat," and low and back in the mouth is the vowel sound found in "bar." I suggest using "aa" or long "a" for the first and "a" for the second. In the middle of the back of the mouth is the vowel sound found in "boar" and the vowel sound found in "boat." I suggest "o" for the first and "ou" for the second (this sound is also always diphthongized; see below). Finally, high in the back are the vowel sounds found in "foot" and "boot." I suggest "u" for the first and "uu" or long "u" for the second.
Please note that we don't all pronounce these words the same way, as there are regional variations and additional vowel sounds, used regionally, in between these sounds. However, I think that a little experience with the new spelling will allow people to accommodate their regional differences without changing the spelling of very many words. And I also think that we should be more tolerant to variations in spelling than has been the case with standard English.
As a personal note, since I am from Western Pennsylvania, I have trouble distinguishing /o/ from /a/ because I use a sound halfway between the two. The first is described as mid-back, tense, round, and open; the second as low back, tense, and unround.
There are also four diphthongs -- combinations of two vowels -- used in English, found in "bite," "bough," "boy," and "few." Also the long "e" and long "o," which I have already mentioned, found in "bay" and "blow," are nearly always diphthongized. These sounds can be represented by "ai," "au," "oi," "iu," "ei," and "ou."
One final change. Often in English a vowel letter is used when there is no sound. In these cases, no letter will be supplied. A number of English consonants do not require a vowel sound to accompany them when occurring at the end of a word, including "d" as in "called," "t" as in "walked," "n" as in "certain," "l" as in "little," and "r" as in "butter."
A List of Vowels and Consonants in Old Spellings and New
|
Letter
|
Old Spelling
|
New Spelling
|
|
a
|
father, bazaar, hurrah, calm, sergeant, hearth
|
faqr, b^zar, h^ra, kam (kalm), sardjnt, harq
|
|
long a
|
rat, ma'am, actor, diaphragm, plaid
|
raat, maam, aaktr, dai^fraam, plaad
|
|
ai
|
aye, stein, height, eye, ice, tie, I, buy, lye
|
ai, stain, hait, ai, ais, tai, ai, bai, lai
|
|
au
|
out, bough, sow (the animal)
|
aut, bau, sau
|
|
b
|
butter, robber
|
b^tr, rabr
|
|
c
|
ocean, machine, special, sure, schist, conscience, shoot, mansion, tissue, nation
|
oucn, m^ciin, specl, cur, cist, kancins, cuut, maanc^n, ticiu, neic^n
|
|
d
|
dead, ladder, made, called, should
|
ded, laadr, meid, kald, cud
|
|
dj
|
graduate, judgment, bridge, soldier, sage, exaggerate, magic
|
graadjuu^t, djudjmnt, bridj, soldjr, seidj, egzaadj^reit, maadjik
|
|
^
|
about, ernest, easily, parliament, son, gallop, flood, tough, circus, but, martyr
|
^baut, ^rn^st, iiz^li, parl^mnt, s^n, gaal^p, fl^d, t^f, sirk^s, b^t, mart^r
|
|
e
|
any, chair, says, rebel, leather, their, leopard, friend, bury, guest
|
eni, tcer, sez, rebl, leqr, qer, leprd, frend, beri, gest
|
|
ei
|
ate, bait, gauge, stay, suede, steak, matinee, feign, sleigh, obey
|
eit, beit, geidj, stei, sweid, steik, maatinei, fein, slei, oubei
|
|
f
|
fight, ruffle, tough, half, physical
|
fait, r^fl, t^f, haaf, fizikl (fiz^kl)
|
|
g
|
grab, egg, ghost, guest, catalogue
|
graab, eig (eg), goust, gest, kaat^log
|
|
h
|
hurry, who, where (for some people)
|
h^ri, huu, hwer
|
|
i
|
damage, England, been, counterfeit, carriage, women, busy
|
daamidj, Ixlnd (Ixglnd), bin, kaunt^rfit, keridj, wim^n, bizi
|
|
long i
|
Caesar, easy, equestrian, see, receive, receipt, people, key, machine, field, debris, amoeba
|
Siizr, iizi, iikwestri^n, sii, risiiv, risiit, piipl, kii, m^ciin, fiild, d^brii, ^miib^
|
|
iu
|
beauty, Euclid, feudal, fewer, view, useful, clue, queue, yew, you, yule
|
biuti, Yiuklid, fiudl, fiuw^r, viu, iusful, kliu, kyiu, yiu, yiu, yiul
|
|
j
|
garage, measure, division, azure
|
g^raj, meijr (mejr), d^vijn, aaj^r
|
|
k
|
cat, raccoon, character, rack, acquainted, lacquer, biscuit, loch, break, talk, Iraq, quest
|
kaat, raakuun, keraaktr (ker^ktr), raak, ^kweintid, laak^r, biskt, lak, breik, tok, ^rak, kwest
|
|
l
|
liver, smile, ball, they'll, isle
|
liv^r, smail, bal, qeil, aul (ail)
|
|
m
|
paradigm, calm, measure, I'm, limbs, Rome, stammer, hymn
|
per^daim, kam (kalm), meijr (mejr), aim, limz, Roum, staamr, him
|
|
n
|
gnat, knight, mnemonic, never, bone, funny, pneumatic
|
naat, nait, niumanik, nevr, boun, funi, niumaatik
|
|
o
|
wash, war, almost, walk, loss
|
wosh, wor, olmoust, wok, los
|
|
oi
|
Freud, boil, Iroquois, toy, buoy
|
Froid, boil, Ir^kwoi, toi, boi-ii
|
|
ou
|
beau, yeoman, sew, wrote, boat, toe, oh, yolk, brooch, depot, owe
|
bou, youmin, sou, wrout, bout, tou, ou, youk, broutc, diipou, ou
|
|
p
|
pink, stepping
|
pink, stepix
|
|
q
|
this, that, breathe, breath
|
qis, qaat, briiq, breq
|
|
r
|
rat, sure, they're, rhythm, carrot, wrought
|
raat, cur, qeir (qeier), riqm, kerit (ker^t), rot
|
|
s
|
certain, nice, psycho, see, it's, scenic, schism, house, loss
|
sertn, nais, saiko, sii, its, siinik, skism, haus, los
|
|
t
|
doubt, yacht, walked, fright, time, kite, thyme, rotten
|
daut, yat, wokt, frait, taim, kait, taim, ratn
|
|
tc
|
cello, cheer, niche, catch, righteous, question, natural
|
tcelou, tcir, nitc, kaatc (ketc), raitc^s, kwesctn, naatcrl
|
|
u
|
wolf, book, should, full
|
wuf (wulf), buk, cud, ful
|
|
long u
|
maneuver, brew, lieu, move, canoe, smooth, rue, fruit
|
m^nuuv^r, bruu, luu, muuv, k^nuu, smuuq, ruu, fruut
|
|
v
|
of, Stephen, vote, have, I've
|
^v, Stiivn, vout, haav, aiv
|
|
w
|
choir, ouija, quick, well, where
|
kwair, wiidj^, kwik, wel, wer (hwer)
|
|
x
|
rink, bring, tongue
|
rixk, brix, t^x
|
|
y
|
Euclid, uniform, hallelujah, yesterday
|
Yiuklid, yiuniform, haaleiluuy^ (haal^luuy^, yest^rdei
|
|
z
|
has, who's, raise, scissors, anxiety, zodiac, brazen, dazzling
|
haaz, huuz, reiz, sizrz, aaxzai^ti, zoudiiaak, braizn, daazllix
|
What does this look like as text, and how easy is it to read? Here is a sample:
We hold these truths to be self-evident: that all men are created equal; that they are endowed by their creator with certain unalienable rights; that among these are life, liberty, and the pursuit of happiness.
Wii hold qiiz truqs t^ bii self-ev^dnt: qaat ol men ar kriieitd iikwl; qaat qei ar indaud bai qer kriieitr wiq s^rtn ^neilii-in^bl raits; qaat ^m^x qiiz ar laif, lib^rti, aand q^ p^rsuut ^f haapiinis.
Our recommendation is that we teach this new method of spelling to all students, that we transcribe or write books for them using this spelling and have them write in this fashion, starting at the lowest grades, and that we use both methods of spelling on all signs and labels in the New World. We think that all of our students should also learn the old method of spelling to some extent, as they will need to read imported books. And we think that adults should be encouraged to learn to read and write following the new method. However, we suggest that the old spelling be very gradually phased out, so those who know only the old method of spelling won't find themselves suddenly unable to find anything to read.
Numeration
by Dan Hopkins
Most of you have been students of mine and have studied different number systems, so you will be familiar with what I have to say. For the benefit of those who have never heard these ideas before, I will explain carefully, but I will keep my explanation short.
While various counting systems have existed in the past, the vast majority of the peoples of the earth have counted by tens. Does this mean that a ten-based system is naturally superior? No. Then why do they count by tens? The answer is very simply that all humans have ten fingers, and it is very easy to count things using your fingers, which are always on you. I had to teach many in this room to not use their fingers when they were little.
In counting by tens on paper, you write one through nine, and then, to indicate ten, you write one and a zero. Although we have all learned that one-zero means "ten," it means "two" in the base two system, it means "three" in the base three system, and so on. Any number could be used as the base for a counting system, and all math problems work correctly in all bases, but this does not mean that all bases are equally good.
Counting by tens is both a good and bad system. It is good because half of the numbers in the system are recognizably dividable by two which we need half of the time, and it is bad because the only other divisor is five, which we need only one fifth of the time. A better base would give us more divisors or at least be a multiple of three rather than a multiple of five.
If there is a system superior to counting by tens, why has it not appeared? And the answer is that it has, and we use it every day. The baker sells donuts by the dozen, soft drinks are packed by the dozen, the clock has twelve hours, the year has twelve months, and the foot has twelve inches. In the past, the pound was twelve ounces, and the mile was also dividable into twelve equal parts. We have a word for a dozen dozen (a gross) and for a dozen times that (a great gross).
Why did people use dozens when they already had a system based on ten? The reason is simple: math is easier when you count by twelves. Tens only allow even divisions by two and five, but twelves allow even divisions of two, three, four, and six.
Think of it this way: we have words we use every day for multiplying by two, three, and four -- doubling, tripling, and quadrupling, but although we have a word for multiplying by five, we don't ever use it. Also, we commonly use words for dividing by two, three, and four -- halving, bisecting, trisecting, and quartering, but we don't even have a word for dividing by five. Doesn't this suggest that dividing by three and four is more important than dividing by five?
In base ten, we have numbers that we can immediately recognize as being divisible by two. We call them "even numbers," and numbers that can't be divided by two which we call "odd numbers." In base twelve, we have the same even numbers plus the number ten, which we can represent with a "T," and the same odd numbers plus the number eleven, which we can represent with an "E," but we also have numbers that we can immediately recognize as divisible by three, and numbers we can immediately recognize as divisible by four. We might call these numbers trine numbers and quad numbers. This means that if you see only the last digit in a long number in base ten, that you can immediately tell if it is an even number or divisible by five, but in base twelve, you can immediately tell if it is even, trine, quad, or divisible by six. For example, let's suppose we have a really long number which ends in three. In the base ten system, do we know if it is divisible by three? No, there's only one chance in three that it is. In the base twelve system, is a number ending in three divisible by three? Always. Here is a chart of all the final digits in base ten and twelve, showing what the possible divisors are:
|
#
|
Base Ten Divisors
|
Base Twelve Divisors
|
|
0
|
two and five
|
two, three, four, and six
|
|
1
|
prime or multiple of primes
|
prime or multiple of primes
|
|
2
|
two
|
two
|
|
3
|
prime or multiple of primes
|
three
|
|
4
|
two
|
two and four
|
|
5
|
five
|
prime or multiple of primes
|
|
6
|
two
|
two and three
|
|
7
|
prime or multiple of primes
|
prime or multiple of primes
|
|
8
|
two
|
two and four
|
|
9
|
prime or multiple of primes
|
three
|
|
T
|
not applicable
|
two
|
|
E
|
not applicable
|
prime or multiple of primes
|
Now how can we evaluate these differences mathematically? Each of the number endings occurs equally often, so we'll count those the same, except each number ending counts 1/10 of the time in base ten and 1/12 of the time in base twelve; however, the numbers used for divisions occur in inverse proportions, 1/2, 1/3, and so on, so we'll score them that way (the periods used below are intentional and will be explained later):
|
#
|
Base Ten Score
|
Base Twelve Score
|
|
0
|
1/2 + 1/5 = 7/10.
|
1/2 + 1/3 + 1/4 + 1/6 = 15./12.
|
|
1
|
|
|
|
2
|
1/2 = 5/10.
|
1/2 = 6/12.
|
|
3
|
|
1/3 = 4/12.
|
|
4
|
1/2 = 5/10.
|
1/2 + 1/4 = 9/12.
|
|
5
|
1/5 = 2/10.
|
|
|
6
|
1/2 = 5/10.
|
1/2 + 1/3 = 10./12.
|
|
7
|
|
|
|
8
|
1/2 = 5/10.
|
1/2 + 1/4 = 9/12.
|
|
9
|
|
1/3 = 4/12.
|
|
10.
|
not applicable
|
1/2 = 6/12.
|
|
11.
|
not applicable
|
|
|
TOTALS
|
29./10./10. = 2.9/10. = .29
|
63./12./12. = 5.25/12. = .4375
|
By these calculations, base twelve is half again as good as base ten.
Now let's look at fractions and decimals. A good numbering system should easily convert fractions to simple decimals (or with base twelve, perhaps we ought to say duodecimals, but I will stick to the easier term). Here again, base twelve beats base ten. Let's do the chart first and the explanation afterwards:
|
Fraction
|
Base Ten Decimal
|
Immediate Divisors
|
Base Twelve Decimal
|
Immediate Divisors
|
|
1/2
|
.5
|
divisible by 5
|
;6
|
divisible by 2, 3 or 6
|
|
1/3
|
.3333...
|
repeating
|
;4
|
divisible by 2 or 4
|
|
1/4
|
.25
|
divisible by 5
|
;3
|
divisible by 3
|
|
1/5
|
.2
|
divisible by 2
|
;2497...
|
repeating
|
|
1/6
|
.1666...
|
repeating
|
;2
|
divisible by 2
|
|
1/7
|
.1428...
|
repeating
|
;186T...
|
repeating
|
|
1/8
|
.125
|
divisible by 5
|
;16
|
divisible by 2,3 or 6
|
|
1/9
|
.1111...
|
repeating
|
;14
|
divisible by 2 or 4
|
|
1/10.
|
.1
|
must add decimal
|
;1249...
|
repeating
|
|
1/11.
|
.09090...
|
repeating
|
;1111...
|
repeating
|
|
1/12.
|
.08333..
|
repeating
|
;1
|
must add decimal
|
This list should extend all the way through the number system for both bases, but the importance of the fractions decreases in proportion with their size anyway. There is a problem in making a comparison in that we have several kinds of decimals, including single, double, and triple digit numbers plus repeating numbers. However, base twelve has five single digit decimals in this list while base ten has only three, and base twelve has two double digit decimals while base ten has only one. Base ten has six repeating numbers while base twelve has only four; however, three of the four repeaters in base twelve repeat only after twelve digits, while only one of the base ten repeaters repeats after ten digits. If you try to divide these decimals, you find that base ten has only four possible divisions without adding additional digits while base twelve has twelve.
Base ten system does pick up an accidental benefit from nine being one less than ten; this makes 1/9 a short-term repeating decimal and therefore all multiples of three a short-term repeating decimal. Base twelve gets a similar benefit for eleven, but eleven is not a multiple for as many numbers, so it's much less of a benefit.
Some other interesting facts: In base ten, prime numbers can end only in 1, 3, 7, and 9, while in base twelve, they can end only in 1, 5, 7, and E. This may make them seem equal, but 2/5ths of base ten numbers might be prime, but only 1/3 of base twelve, so primes are easier to spot. In addition, whenever you square a prime number, the last digit is always 1 in base twelve, but it could be 1 or 9 in base ten. Other squares of numbers are also more easily identified in base twelve, as base ten has six final digits for squares (1, 4, 9, 6, 5, and 0) while base twelve has only four (1, 4, 9, and 0).
What about the difficulty of learning the multiplication table? Here, the advantage is also with base twelve. With base ten, there are a hundred multiplications to learn, but the one's and zero's are obvious, thus sixty-four remain. Of these, the two's and five's are extremely easy, so there are really just thirty-six difficult numbers to learn. With base twelve, there are a hundred and forty-four multiplications to learn, but the one's and two's can be subtracted, leaving a hundred to learn. Of these, the two's, three's, four's, sixes, eight's, and nine's are easy, leaving just sixteen difficult numbers to learn.
Why are two's, three's, four's, sixes, eight's, and nine's easy in base twelve? Here's how they count, looking just at the final digit:
Two's: 2, 4, 6, 8, T, 0
Three's: 3, 6, 9, 0
Four's: 4, 8, 0
Sixes: 6, 0
Eight's: 8, 4, 0
Nine's: 9, 6, 3, 0
Base Ten Multiplication Table
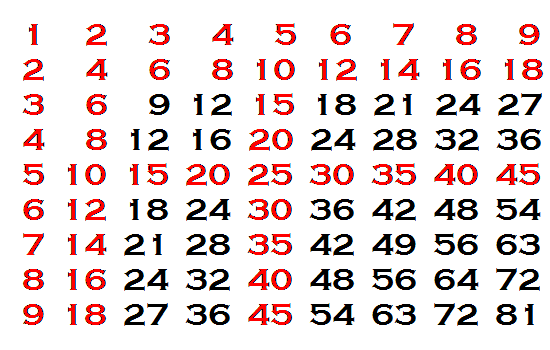
Base Twelve Multiplication Table
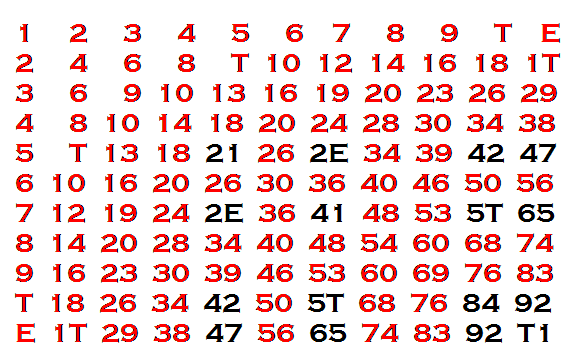
It must be admitted that each of the difficult numbers to learn in base twelve has a larger number of options (twelve rather than ten) each time, but this disadvantage is recovered, however, by base twelve numbers having somewhat fewer digits (123,456,789. = 35,418,T99;).
Besides making math simpler, base twelve also makes stacking easier. With base ten, there is a choice between two stacks of five or five stacks of two. With base twelve, there can be two stacks of six, three stacks of four, four stacks of three, or six stacks of two. Finally, only with base twelve is a three-dimensional stack possible, one of two by two by three.
Two questions are likely to pop up at this point, How do you distinguish between base ten numbers and base twelve numbers, since they look just alike? And, How do you pronounce base twelve numbers?
Base ten numbers are identifiable because they use a period to mark the units place. We decided to indicate base twelve numbers in the same way with a semicolon. In a circumstance where this method could cause confusion, you can also indicate them with a following "tn" and a following "dz," thus 100tn and 100dz.
Here's how you count using base twelve, skipping the obvious: nine, ten, eleven, twelve, dozen one, dozen two, dozen three, ... dozen nine, dozen ten, dozen eleven, two dozen, two dozen one, two dozen two, etc.
English has a word for a dozen dozen, "gross," and a dozen times that, "great gross." We thought our own words would sound better. Here's a partial list:
|
10;
|
dozen
|
|
100;
|
grand
|
|
1,000;
|
myriad
|
|
10,000;
|
twelve myriad
|
|
100,000;
|
grand myriad
|
|
1,000,000;
|
durmir
|
|
1,000,000,000;
|
twamir
|
|
1,000,000,000,000;
|
katmir
|
|
|
|
|
1/10;
|
twelth (intentional change)
|
|
1/100;
|
granth
|
|
1/1,000;
|
mirth
|
|
1/10,000;
|
twelth mirth
|
|
1/100,000;
|
granth mirth
|
|
1/1,000,000;
|
durmirth
|
|
1/1,000,000,000;
|
twamirth
|
|
1/1,000,000,000,000;
|
katmirth
|
Our recommendations are that we gradually introduce base twelve into our community, starting with the youngest grades in school. All students would be also required to learn base ten and base two, and be familiarized with other bases and counting systems. Thus base twelve would only gradually replace base ten, causing the minimum amount of disturbance for those who learned to count the old way. Simple base twelve numbers can be calculated using base ten math by anyone not knowing their multiplication table in base twelve, such as three times five dozen, which the new math calls one grand, three dozen, and the old math, fifteen dozen.
Distance, Area, Volume, Weight, Temperature, and Direction
by Dan Hopkins
Before the origin of the metric system in Napoleon's France, there was a debate whether to adopt base twelve or not. The scientists recognized the value of base twelve but thought it unlikely to be adopted by the rest of the world. Therefore, they based their number system entirely on ten, originally including a ten hour day. A metric calendar was also proposed.
If we decide to use base twelve, then we can't use the metric system. However, the English system of weights and measures was originally largely based on twelve, so with a little juggling, the adoption of some new terms, and the redefinition of other terms, we can achieve a totally base twelve system without a great deal of change.
For example, the current English mile is 5,280. feet or 1,760. yards. There have been various miles over the years; for instance, the Roman mile at 4,860. feet or 1,620. yards and the nautical mile at 6080. feet. With a small change in the length of the mile, a reduction from 1,760. yards to 1,728. yards, the length of a mile becomes exactly 1,000; yards, making the following chart possible. Note that "notch" is a new term, "fathom" has been doubled in size, a "rod" has been greatly increased in size, a "furlong" has been much reduced, and a league has been somewhat increased. "Inch," "foot," and "yard" remain exactly the same. The acre has been reduced to approximately half its current area.
twelve notches = one inch = 1/10; foot or 1/12. foot
twelve inches = one foot
three feet = one yard
twelve feet = one fathom = 10; feet or 12. feet
twelve fathoms = one rod = 100; feet or 144. feet
twelve rods = one furlong = 1,000; feet or 1,728. feet
three furlongs = one mile = 3,000; feet or 5,184. feet
four miles = one league = 10,000; feet or 20,736. feet
one square rod = one acre = 10,000; sq. feet or 20,736. sq. feet
nine grand acres = one square mile
For volume measurement, it makes sense to get away from the confusion involved in having apothecaries' fluid measurements, liquid measurements, and dry measurements, and to combine them all into one measurement, which is also related to cubic feet and inches. The US standard gallon is 231. cubic inches, the British 277.42. If we made the US gallon slightly smaller, 216. cubic inches, it would be six inches cubed exactly. This makes a cubic foot exactly eight gallons. All of the following measures have been changed. Their new values make multiplication and division easier and provide lots of container sizes. In addition, the volume can be indicated as cubic inches at all times.
one fluid dram = ;16 cubic inches = .125 cubic inches
two fluid drams = one teaspoon = ;3 cubic inches = .25 cubic inches
six fluid drams = one tablespoon = ;9 cubic inches = .75 cubic inches
twelve fluid drams = one flounce = 1;6 cubic inches = 1.5 cubic inches
three flounces = one gill = 4;6 cubic inches = 4.5 cubic inches
eight flounces = one cup = 10; cubic inches = 12. cubic inches
twelve flounces = four gills = one pint = 16; cubic inches = 18. cubic inches
three pints = one quart = 46; cubic inches = 54. cubic inches
four quarts = one gallon = 160; cubic inches = 216. cubic inches
eight gallons = one bushel = one cubic foot = 1,000; cubic inches = 1,728. cubic inches
twelve gallons = one peck = 1;6 cubic feet = 1,600; cubic inches = 2,592. cubic inches
six bushels = four pecks = one barrel = 6; cubic feet = 6. cubic feet
three barrels = one hogshead = 16; cubic feet = 18. cubic feet
In the metric system, weight and volume are connected through a measurement of water at a given temperature. This connection has no real value. It is proposed instead to use the standard pound weight but to adjust the other values accordingly.
twelve grains = one dram
twelve drams = one ounce
twelve ounces = one pound
twelve pounds = one stone
100; stones or 1,000; pounds = one ton
Temperature presents an interesting problem. Fahrenheit produced a somewhat defective scale, because 0° and 100.° can only be approximated, while freezing and boiling (used on the Celsius scale) can be precisely determined; on the other hand, the Celsius scale is quite compressed, and a degree on it covers nearly twice the range of a Fahrenheit degree. Twenty degrees Celsius is on the cool side; thirty degrees is too hot. A scale with 144. points between 0° and 100;° might help. Let's convert some temperatures to see. All the temperatures on the same line in the following scale are equal.
|
Dozens
|
Fahrenheit
|
Celsius
|
|
0°D
|
32.°F
|
0°C
|
|
10;°D
|
47.°F
|
8.3°C
|
|
20;°D
|
62.°F
|
16.6°C
|
|
30;°D
|
77.°F
|
25.°C
|
|
40;°D
|
92.°F
|
33.3°C
|
|
50;°D
|
107.°F
|
41.7°C
|
|
60;°D
|
122.°F
|
50.°C
|
|
70;°D
|
137.°F
|
58.3°C
|
|
80;°D
|
152.°F
|
66.7°C
|
|
90;°D
|
167.°F
|
75.°C
|
|
T0;°D
|
182.°F
|
83.3°C
|
|
E0;°D
|
197.°F
|
91.7°C
|
|
100;°D
|
212.°F
|
100.°C
|
The results show that the base twelve scale has more values within the comfort range than does the Celsius scale but somewhat fewer than on the Fahrenheit scale. On the base twelve temperature scale, a dozen degrees cover the same range as 15.° on the Fahrenheit scale which is equal to 8.3° on the Celsius scale. Of course, it won't normally be necessary to indicate that you are using the dozen scale; just say it's two dozen and eleven degrees, and the other person will understand.
The final matter is direction. Rather than divide the sphere into 360.°, the base twelve system divides it by twelves. That this is logical is seen by the fact that fighter pilots use o'clock rather than degrees. Thus, one degree by the new system equals 2.5 degrees in the old. The other values have all changed as well. This scale is similar to the time scale, which we will look at later.
twelve ticks = one instant
twelve instants = one second
twelve seconds = one minute
twelve minutes = one degree
twelve degrees = one o'clock
twelve o'clock = one sphere
As an alternate method, Mariner compass directions divided the compass into thirty-two [two dozen and eight] parts, each of which was 11.25° using the old system. With a base twelve compass having a grand degrees, each of these parts is 4;6° (4½°), a more compatible number mathematically (because, without adding decimal places, 11.25 can be evenly divided by only five, while 4;6 can be divided by 2, 3, and 6). It's not necessary to give all 32 points, as the values from north to east will show the pattern of the rest (the more important directions are capitalized and have an abbreviation):
North = twelve o'clock = 0;° = N
north by east = 4;6°
North, Northeast = 9;° = NNE
northeast by north = E;6°
Northeast = 16;° = NE
northeast by east = 1T;6°
East, Northeast = 23;° = ENE
east by north = 27;6°
East = three o'clock = 30;° = E
The Calendar and Time
by Charles Glass
Most early calendars used the moon. It was an obvious choice. The moon was in the sky half of the time, usually visible during the day and unmistakable at night, and the daily difference in the face of the moon could easily be observed, with seven days between phases and a month before the cycle repeated. Using the moon for a calendar has some flaws: The phases of the moon are 7.38 days apart, and the month is 29.53 days long. This means that counting seven days as a week or twelve months as a year is going to quickly produce errors. The days can't match the moon's phases, and the year is eleven days too short, so there's no way to produce agreement. Therefore, except for religious purposes, the lunar calendar gave way to a solar one (the Jewish and Moslem calendars are still lunar calendars). However, the seven day week and month of about four weeks remained.
The calendar we use is the Roman calendar. Julius Caesar changed it considerably in 46 BC, adding a leap day every four years then, and later Pope Gregory modified it a little, removing leap days at the end of centuries, thus it is sometimes called a Julian or Gregorian calendar. Pope Gregory's changes weren't adopted by the English until 1752, when the error had reached eleven days, thus the day after September 2, 1752, was September 14.
There's a problem with the current calendar because dates and days of the week do not correspond from year to year. Everyone knows the day of the year on which they were born, but few people know the day of the week on which they were born, and very few people know which day of the week their birthday will appear on next. Since many stores, businesses, and gas stations are closed on Sundays and sometimes on Saturdays in the US, not knowing the day of the week of some future date can create problems.
To solve the problem of not being able to connect days to dates, a couple of calendars have been suggested, one with 13 months of exactly 28 days each and the other with four seasons of 91 days each, each containing two months of 30 days and one month of 31 days. However, both of these calendars must employ a trick: one day of a non-leap year and two days of a leap year are not counted as days of the week; thus a week might go Sunday, Monday, Leap Day, Tuesday, and so on. This allows every date to fall on the same day of the week every year. However, pressure from three religions has blocked the adaptation of the second calendar, called the World Calendar.
The old calendar can be similarly adapted to a six day week. There are two ways to do this. With the first, the year is divided into five seasons, each six dozen and one days long, the last day not being a day of the week. The problem with this scheme is that a dozen weeks is a long period to try to keep track of. With the second, the year is divided into twelve months, each month having five weeks. Every three months, there is a special day which is not part of the week. With both of these calendars, Leap Year Day, which comes once every four years, is also not part of the week, and with the second, New Year's Day is not part of the week either.
Why would we want a six day week in our calendar? I see three advantages. First, it makes work scheduling much easier for jobs that must be worked every day. Second, it allows easier computation of time, especially since we are moving to base twelve. The day has twelve hours, and there are twelve days and nights in one week, so the week is 100; hours long. The year, with a dozen weeks and five seasons or with a dozen months of five weeks each, has 5,000; hours, not counting the 5¼ extra days (T6; hours) mentioned above, which can be computed back in. Third, it allows the calendar and the clock to be homogenized, so a watch or clock can be made that shows days, weeks, and months as well. I also see only one problem, the same problem faced in moving from the lunar year to the solar year and from the Julian calendar to the Gregorian -- religion.
There are three ways that people can adapt their religious beliefs to the new calendar. One: keep a record of the days of the week in the old calendar or also keep an old calendar, and continue to observe Saturday or Sunday as before. This is what religions did with their holy days (holidays) based on the lunar calendar when the move to the solar calendar occurred. Two: consider the first or last day of the week to be the Sabbath, and just worship God six dozen times a year. Three: get away from the notion that God should be worshipped one particular day a week and instead worship every day. Someone has suggested that this change in the calendar would be an advantage to us simply because it would cause those who are religiously rigid to lose interest in joining us.
If we do adapt the new calendar, I would suggest some additional changes. First, start the year with the solstice, when it originally began. That date is now December 21, due to inaccurate calendars in the past. The significance of this day is that it is the day when the sun begins to rise through the sky again. We now call it the beginning of winter, but during the middle ages, it was considered to be mid-winter. Of all days of the year, it is the most easy to determine by the use of the sun alone. Second, change the names of the days of the week, which are currently a mixture of Norse gods and heavenly bodies. I suggest using the six visible planets, including the Earth, in order of their distance from the sun, that is, Mercury, Venus, Earth, Mars, Jupiter, and Saturn. If there is any doubt about whether we are talking about a planet or a date, we can call them Mercury day, Venus day, Earth day, Mars day, Jupiter day, and Saturn day. Third, if we use months, abandon January-December, which no longer apply, and use the names of the constellations overhead at the time. These are the same names used in the zodiac, but not the same times of the year associated with them. Finally, I would suggest abandoning counting the days of the month, since it will no longer be necessary. Instead of saying "Gemini the thirtieth," which does not indicate the day of the week, say, "the fifth Saturn in Gemini" or "Gemini, Saturn the fifth." Thus, the part of the month and the day of the week will always be mentioned.
Following is a proposed calendar following this scheme, which includes the equivalent dates from the old calendar. Please note that your birthday will forever fall on the same day of the week, and that this calendar is the only one you will ever need.
The Year
New Years' Day (Dec 22)
GEMINI = The Twins
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
D23
|
D24
|
D25
|
D26
|
D27
|
D28
|
|
D29
|
D30
|
D31
|
J1
|
J2
|
J3
|
|
J4
|
J5
|
J6
|
J7
|
J8
|
J9
|
|
J10
|
J11
|
J12
|
J13
|
J14
|
J15
|
|
J16
|
J17
|
J18
|
J19
|
J20
|
J21
|
CANCER = The Crab
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
J22
|
J23
|
J24
|
J25
|
J26
|
J27
|
|
J28
|
J29
|
J30
|
J31
|
F1
|
F2
|
|
F3
|
F4
|
F5
|
F6
|
F7
|
F8
|
|
F9
|
F10
|
F11
|
F12
|
F13
|
F14
|
|
F15
|
F16
|
F17
|
F18
|
F19
|
F20
|
LEO = The Lion
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
F21
|
F22
|
F23
|
F24
|
F25
|
F26
|
|
F27
|
F28
|
M1
|
M2
|
M3
|
M4
|
|
M5
|
M6
|
M7
|
M8
|
M9
|
M10
|
|
M11
|
M12
|
M13
|
M14
|
M15
|
M16
|
|
M17
|
M18
|
M19
|
M20
|
M21
|
M22
|
Spring Day
M23
== In Leap Years Only ==
LEO = The Lion
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
F21
|
F22
|
F23
|
F24
|
F25
|
F26
|
|
F27
|
F28
|
F29
|
M1
|
M2
|
M3
|
|
M4
|
M5
|
M6
|
M7
|
M8
|
M9
|
|
M10
|
M11
|
M12
|
M13
|
M14
|
M15
|
|
M16
|
M17
|
M18
|
M19
|
M20
|
M21
|
Spring Day
M22
Leap Day
M23
=====
VIRGO = The Virgin
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
M24
|
M25
|
M26
|
M27
|
M28
|
M29
|
|
M30
|
M31
|
A1
|
A2
|
A3
|
A4
|
|
A5
|
A6
|
A7
|
A8
|
A9
|
A10
|
|
A11
|
A12
|
A13
|
A14
|
A15
|
A16
|
|
A17
|
A18
|
A19
|
A20
|
A21
|
A22
|
LIBRA = The Scales
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
A23
|
A24
|
A25
|
A26
|
A27
|
A28
|
|
A29
|
A30
|
M1
|
M2
|
M3
|
M4
|
|
M5
|
M6
|
M7
|
M8
|
M9
|
M10
|
|
M11
|
M12
|
M13
|
M14
|
M15
|
M16
|
|
M17
|
M18
|
M19
|
M20
|
M21
|
M22
|
SCORPIO = The Scorpion
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
M23
|
M24
|
M25
|
M26
|
M27
|
M28
|
|
M29
|
M30
|
M31
|
J1
|
J2
|
J3
|
|
J4
|
J5
|
J6
|
J7
|
J8
|
J9
|
|
J10
|
J11
|
J12
|
J13
|
J14
|
J15
|
|
J16
|
J17
|
J18
|
J19
|
J20
|
J21
|
Summer Day
J22
Sagittarius = The Archer
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
J23
|
J24
|
J25
|
J26
|
J27
|
J28
|
|
J29
|
J30
|
J1
|
J2
|
J3
|
J4
|
|
J5
|
J6
|
J7
|
J8
|
J9
|
J10
|
|
J11
|
J12
|
J13
|
J14
|
J15
|
J16
|
|
J17
|
J18
|
J19
|
J2O
|
J21
|
J22
|
CAPRICORN = The Goat
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
J23
|
J24
|
J25
|
J26
|
J27
|
J28
|
|
J29
|
J30
|
J31
|
A1
|
A2
|
A3
|
|
A4
|
A5
|
A6
|
A7
|
A8
|
A9
|
|
A10
|
A11
|
A12
|
A13
|
A14
|
A15
|
|
A16
|
A17
|
A18
|
A19
|
A20
|
A21
|
AQUARIUS = The Water Boy
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
A22
|
A23
|
A24
|
A25
|
A26
|
A27
|
|
A28
|
A29
|
A30
|
A31
|
S1
|
S2
|
|
S3
|
S4
|
S5
|
S6
|
S7
|
S8
|
|
S9
|
S10
|
S11
|
S12
|
S13
|
S14
|
|
S15
|
S16
|
S17
|
S18
|
S19
|
S20
|
Fall Day
S21
PISCES = The Fish
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
S22
|
S23
|
S24
|
S25
|
S26
|
S27
|
|
S28
|
S29
|
S30
|
O1
|
O2
|
O3
|
|
O4
|
O5
|
O6
|
O7
|
O8
|
O9
|
|
O10
|
O11
|
O12
|
O13
|
O14
|
O15
|
|
O16
|
O17
|
O18
|
O19
|
020
|
O21
|
ARIES = The Ram
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
022
|
023
|
024
|
O25
|
026
|
027
|
|
028
|
029
|
030
|
O31
|
N1
|
N2
|
|
N3
|
N4
|
N5
|
N6
|
N7
|
N8
|
|
N9
|
N10
|
N11
|
N12
|
N13
|
N14
|
|
N15
|
N16
|
N17
|
N18
|
N19
|
N20
|
TAURUS = The Bull
|
Mercury
|
Venus
|
Earth
|
Mars
|
Jupiter
|
Saturn
|
|
N21
|
N22
|
N23
|
N24
|
N25
|
N26
|
|
N27
|
N28
|
N29
|
N30
|
D1
|
D2
|
|
D3
|
D4
|
D5
|
D6
|
D7
|
D8
|
|
D9
|
D10
|
D11
|
D12
|
D13
|
D14
|
|
D15
|
D16
|
D17
|
D18
|
D19
|
D20
|
Winter Solstice
D21
NOTE: Those born during the few days that vary between the two calendars from year to year in Leo and March do not change their birthday every leap year. If born during the leap year, they would use the leap year date, which is the same every year, and if born during one of the other three years, they would always use the regular date. The only person having to change days would be the person born on Leap Day, which happens with both calendars.
One other important consideration in adopting base twelve and a new calendar: We have to start numbering the years by another system, as decades, centuries, and millenium are incompatible. I suggest that we begin at approximately the time that the New World was discovered and on the solstice at that time. Thus, December 21, 1964, will be the beginning of year one in our calendar. I suggest, for events happening before that date, that we continue to use the Julian/Gregorian, base ten method of reckoning years, so the dates will be visually recognizable. For events happening in the old world after that date, I suggest we supply both dates side by side. And for events in the New World, I suggest using our new calendar only.
A small matter to consider is the problem of leap days. The leap day overcompensates for the length of the day by eleven minutes ten seconds. This adds up to a full day every 129. years. In order to make up the difference, we need to drop a leap day every now and then. According to the current scheme, a complex method of making these changes has been adopted -- not counting a leap day at the end of most centuries and counting them at the end of others. I suggest dropping every thirty-second (two dozen and eighth) leap day, which will keep the calendar accurate for myriads of years.
Finally, in order to divide our time by twelve, we are going to have to change our clocks and watches, but by less than you think. The first hand takes twelve hours to make a revolution, the second takes one hour to make a revolution, and the third takes one minute to make a revolution, thus the first two hands are already base twelve, while the third is not, so any watch or clock with just two hands can be converted simply by changing the clock face. In fact, if the second hand moves smoothly and the distance between the numbers of the clock are divided into twelve parts, the second hand can take the place of the third hand for most uses.
twelve instants = one second = 2.1 seconds on the old clock
twelve seconds = one minute = 25. seconds on the old clock
twelve minutes = one moment = five minutes on the old clock
twelve moments = one hour
twelve hours = one day or one night
six days and six nights = one week
five weeks = one month
twelve months = one year
twelve years = one dozade
As I said in discussing the calendar, a clock can be designed to indicate the year, month, week, and day as well, and this just requires hands which move slower than the hour hand and marking these periods around the edge. Adjustment would have to be made for the extra days, however, either through manual adjustment or through a mechanism within the clock.
This concludes the initial reports recommending changes in spelling, numbering, weights, measures, directions, the calendar, and timekeeping. These changes and others were finally adopted by a vote of the Community within the New World on Earth the second, Taurus, year three.
|
